Master's Thesis
QCD corrections to Higgs production in association with vector bosons at the LHC
Abstract
The total inclusive hadronic cross section for the production of a Higgs boson in association with a massive vector boson at proton-proton-colliders like the Large Hadron Collider at next-to-leading
order (NLO) in the quantum chromodynamics (QCD) coupling constant is being calculated.
This computation is conducted in perturbative QCD in the framework of the Standard Model.
To determine the NLO corrections, the entire calculation is performed in arbitrary space-time dimensions with $\stdim = 4 – 2 \vardim$ by using conventional dimensional regularization. Ultra-violet and parton distribution function (PDF) renormalization are carried out in the modified minimal subtraction ($\msbar$) scheme.
Throughout the course of this thesis, $\Nf = 5$ light quark flavors are assumed which are treated as massless and the effects of the top quark are neglected.
The computation is facilitated by factorizing the amplitude into a Drell-Yan-like production process for a virtual massive vector boson and a part describing its decay into a real vector boson and a Higgs particle.
It is recognized that the QCD corrections only apply to the production process.
Therefore, our final result essentially coincides with the corresponding Drell-Yan corrections at that order and also agrees with other findings in the literature.
Various $\gamma_5$ schemes for the $\stdim$-dimensional $\gamma_5$ matrices appearing in the intermediate steps due to the weak vector bosons are applied and it is observed that all schemes eventuate in the same final result in the limit $\stdim \rightarrow 4$.
In addition, explanations are given on how the final formula for the NLO QCD cross section to our process can numerically be integrated using Monte Carlo integration techniques and how the impact of the radiative corrections to the leading order result is quantified with the appropriate $K$-factors.
It will also be addressed how the theoretical uncertainty of our prediction can be conventionally estimated by exploring its dependence on the renormalization and factorization scales and on the choices and errors of the various available PDF parametrizations.
Download
Contents
1 Introduction 5
1.1 Outline . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 8
2 The Standard Model and Electroweak Symmetry Breaking 10
2.1 Mathematical formulation of ’the’ Standard Model . . . . . . . . . . . . . . . . . . . . 10
2.2 Feynman rules in momentum space . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 15
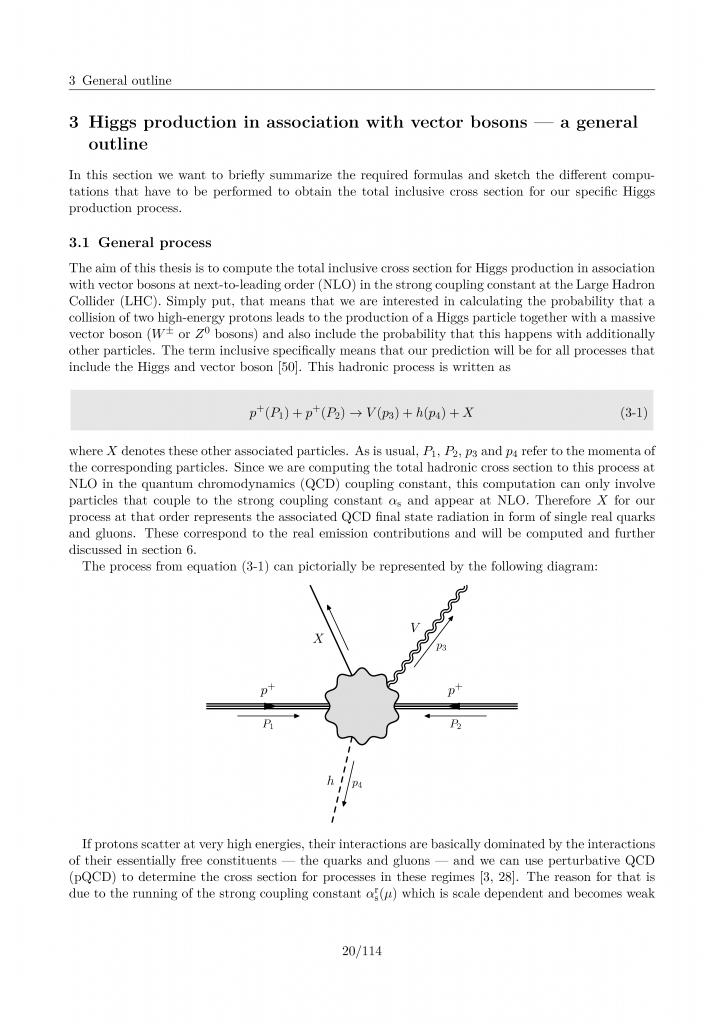
3 General outline 20
3.1 General process . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 20
3.2 General approach . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 22
3.3 Born cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 22
3.4 Virtual gluon corrections at NLO . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 24
3.5 Real emission contributions at NLO . . . . . . . . . . . . . . . . . . . . . . . . . . . . 25
4 Born cross section 27
4.1 Feynman amplitude . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 28
4.2 Squared, unpolarized Feynman amplitude . . . . . . . . . . . . . . . . . . . . . . . . . 29
4.3 Dimensional regularization schemes and the
5 problem . . . . . . . . . . . . . . . . . 32
4.4 Two-body phase space . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 36
4.5 Total partonic cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 38
4.6 Total hadronic cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 41
5 Virtual gluon corrections at NLO 43
5.1 Feynman amplitude . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 45
5.2 Evaluation of the vertex form factor . . . . . . . . . . . . . . . . . . . . . . . . . . . . 46
5.3 Performing the loop integral . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 50
5.4 NLO virtual correction to the squared, unpolarized Feynman amplitude . . . . . . . . 55
5.5 Total partonic cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 57
5.6 Summary, general picture and outlook . . . . . . . . . . . . . . . . . . . . . . . . . . . 57
6 Real emission contributions at NLO 59
6.1 Real gluon emission contributions at NLO . . . . . . . . . . . . . . . . . . . . . . . . . 61
6.1.1 Feynman amplitude . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 62
6.1.2 Squared, unpolarized Feynman amplitude . . . . . . . . . . . . . . . . . . . . . 63
6.1.3 Review on kinematics . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 66
6.1.4 Gluon parametrization . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 69
6.1.5 Soft and collinear divergences . . . . . . . . . . . . . . . . . . . . . . . . . . . . 71
6.1.6 Three-body phase space decomposition . . . . . . . . . . . . . . . . . . . . . . 74
6.1.7 Total partonic cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 77
6.2 Real quark and antiquark emission contributions at NLO . . . . . . . . . . . . . . . . 81
6.2.1 Crossing relations and squared, unpolarized Feynman amplitude . . . . . . . . 81
6.2.2 Collinear divergence . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 83
6.2.3 Total partonic cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 84
6.3 Summary and general picture . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 86
7 Total partonic and hadronic cross section at NLO 89
7.1 PDF contributions at NLO . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 89
Contents
7.2 Pole cancellations and total partonic cross section at NLO . . . . . . . . . . . . . . . . 90
7.3 Total hadronic cross section at NLO . . . . . . . . . . . . . . . . . . . . . . . . . . . . 94
8 Conclusion 96
8.1 Summary, numerical evaluation and theoretical uncertainty estimation . . . . . . . . . 96
8.2 Outlook and suggestions for extended investigations . . . . . . . . . . . . . . . . . . . 98
A Alternative derivation via full factorization of the cross section 99
A.1 Hierarchical phase space decomposition . . . . . . . . . . . . . . . . . . . . . . . . . . 100
A.2 Factorization of the cross section . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 101
A.3 Re-derivation of the partonic Born cross section . . . . . . . . . . . . . . . . . . . . . . 103
A.4 Re-derivation of the partonic real gluon emission contributions at NLO . . . . . . . . . 104
Acknowledgements 106
List of Acronyms 107
References 109